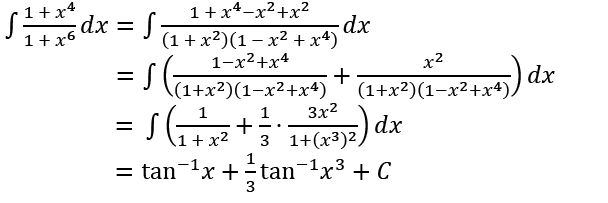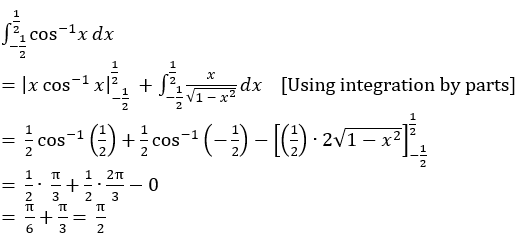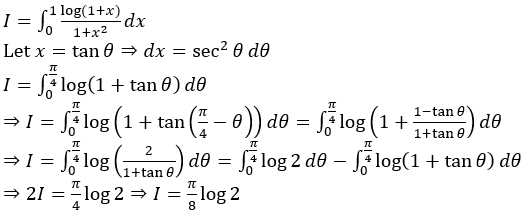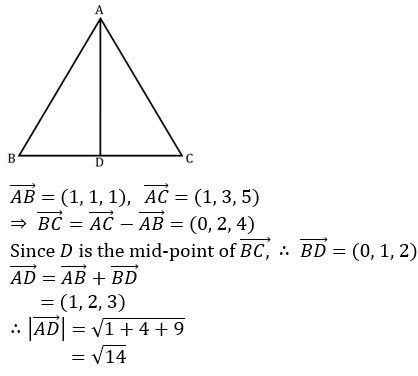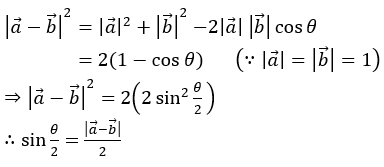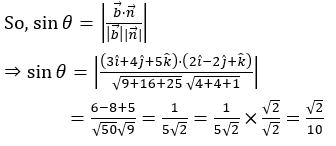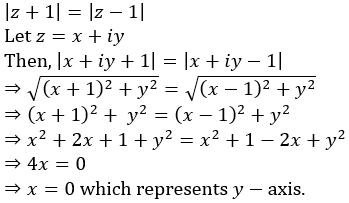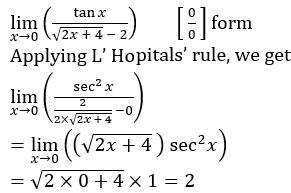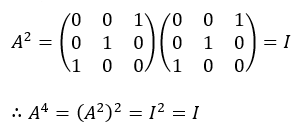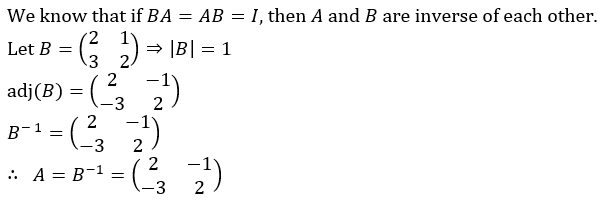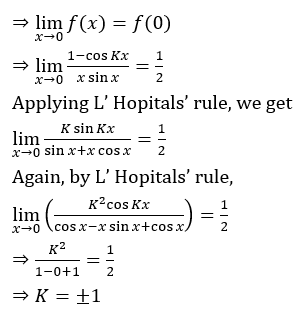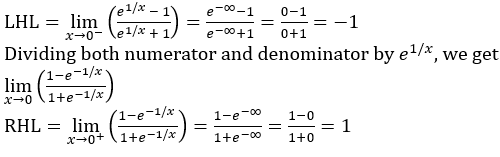KCET 2020 MATHEMATICS QUESTION PAPER

The Karnataka Common Entrance Test (KCET) is an entrance examination conducted by the Karnataka Examination Authority (KEA) for admission to various undergraduate courses in the state of Karnataka. The mathematics section of the KCET exam is an essential component for students aspiring to pursue engineering, architecture, and other related fields.

Overview of the KCET Exam

KCET is a state-level entrance exam held annually for admission to undergraduate courses in engineering, pharmacy, agriculture, and other disciplines offered by colleges in Karnataka. It serves as a gateway for students to secure seats in prestigious institutions across the state.

Importance of Mathematics Section in KCET

It evaluates their understanding of mathematical concepts, problem-solving abilities, and analytical thinking skills.

Format and Structure of KCET 2020 Mathematics Paper

The paper was divided into different sections, each focusing on specific mathematical concepts.

Difficulty Level of the Questions

The questions in the KCET 2020 Mathematics paper ranged from moderate to challenging, aiming to assess candidates’ depth of knowledge and conceptual clarity.

Key Topics Covered in the Mathematics Section

Algebra

Algebraic topics included equations, inequalities, polynomials, sequences, and series.

Trigonometry

Trigonometric functions, identities, equations, and their applications were covered extensively.

Calculus

Calculus topics encompassed limits, derivatives, integrals, and their applications in solving real-world problems.

Geometry

Geometry questions involved concepts related to lines, angles, triangles, circles, and solid figures.

KCET 2020 MATHEMATICS QUESTION PAPER

1. If

$y = 2 x^{n + 1} + \frac{3}{x^{n}}$

, then
$x^{2} \frac{d^{2} y}{d x^{2}}$
is

a. y
b. 6n(n + 1)y
c. n(n +1 )y
d.
$x \frac{d y}{d x} + y$

Solution:

Answer: (c)

y= (2x)⁽ⁿ⁺¹⁾ +(3x)^(-n)

⇒ dy/dx = 2(n+1)xⁿ-(3nx)^(-n-1)

⇒ (d² y)/(dx²) = 2n(n+1)x^(n-1)+3n(n+1)x^(-n-2)

⇒ x² (d² y)/(dx²) = n(n+1) [(2x)⁽ⁿ⁺¹⁾+3/xⁿ ]

⇒ x² (d² y)/(dx²) = n(n+1)y

2. If the curves are 2x = y² and 2xy = K intersect perpendicularly, then the value of K² is

a. 8
b. 4
c. 2√2
d. 2

Solution:

Answer: (a)

2x = y² . . . (1)

2xy = K . . . (2)

Solving (1) and (2), we get

(x,y) = (K^(2/3)/2, K^(1/3))

Differentiating (1) and (2) w.r.t. x

m₁ = dy/dx = 1/y …(3)

m₂ = dy/dx = -y/x …(4)

∵ Both curves intersect each other perpendicularly

∴m₁ m₂ = -1

⇒ -1/x = -1

⇒ x = 1

⇒ K^(2/3)= 2

⇒ K² = 8

3. If (xe)^y = e^x, then dy/dx is

a.
$\frac{e^{x}}{x (y - 1)}$
b.
$\frac{l o g x}{(1 + l o g x)^{2}}$
c.
$\frac{1}{(1 - l o g x)^{2}}$
d.
$\frac{l o g x}{1 + l o g x}$

Solution:

Answer: (b)

(xe)^y = e^x

⇒y (log x + 1) = x

⇒ y = x/(logx+1)

∴
$\frac{d y}{d x} = \frac{l o g x}{(l o g x + 1)^{2}}$

4. If the side of a cube is increased by 5%, then the surface area of a cube is increased by

a. 20%
b. 10%
c. 60%
d. 6%

Solution:

Answer: (b)

Let one side of the cube be x and surface area be A

So, dx = 5% = 5x/100

Then, A = (6x)²

⇒dA/dx = 12x

⇒dA = (12x)dx

⇒dA = (12x) (5x/100)

⇒dA = 10A/100

⇒dA = 10%

5. The value of

$\int \frac{1 + x^{4}}{1 + x^{6}} d x$

a. tan^-1x +(1/3)tan^-1x² + C
b. tan^-1 x+tan^-1x³+C
c. tan^-1x+(1/3)tan^-1 x³+C
d. tan^-1x-(1/3)tan^-1 x³+C

Solution:

Answer: (c)

6. The maximum value of

\frac{l o g_{e} x}{x}

a. -1/e
b. e
c. 1
d. 1/e

Solution:

Answer: (d)

$y = \frac{l o g_{e} x}{x}$

⇒
$\frac{d y}{d x} = \frac{1 - l o g_{e} x}{x^{2}}$

For maxima, dy/dx = 0

⇒ 1 – log_e x = 0

⇒ x = e

dy/dx changes sign from positive to negative at x = e

∴ y_max = 1/e

7. The value of

$\int e^{s i n x} s i n 2 x d x$

a. 2e^{sin x}(cosx-1)+C
b. 2e^{sin x}(sinx-1)+C
c. 2e^{sin x}(sinx+1)+C
d. 2e^{sin x}(cosx+1)+C

Solution:

Answer: (b)

$I = \int e^{s i n x} s i n 2 x d x = 2 \int e^{s i n x} s i n x c o s x d x$

Let t =sinx

⇒ dt=cosx dx

Therefore, I = 2∫te^t dt

= 2(te^t – e^t) + C

= 2(sinx – 1)e^{sin x} + C

8. The value of

$\int_{- 1 / 2}^{1 / 2} c o s^{- 1} x d x$

a. π²/2
b. π
c. π/2
d. 1

Solution:

Answer: (c)

9. If

\int \frac{3 x + 1}{(x - 1) (x - 2) (x - 3)} d x = A l o g | x - 1 | + B l o g | x - 2 | + C l o g | x - 3 | + C

, then the values of A, B and C are respectively,

a. 2, -7, 5
b. 5, -7, -5
c. 2,-7, -5
d. 5, -7, 5

Solution:

Answer: (a)

Now, 3x + 1 = A(x-2)(x-3) + B(x-1)(x-3) + C(x-1)(x-2) [From eqn. (1)]

Putting x = 1, x = 2, x = 3 in the above equation one at a time, we get

A = 2, B = -7, C = 5.

10. The value of

$\int_{0}^{1} \frac{l o g (1 + x)}{1 + x^{2}} d x$

a. (π/8)log2
b. (π/2)log 2
c. (π/4)log 2
d. 1/2

Solution:

Answer: (a)

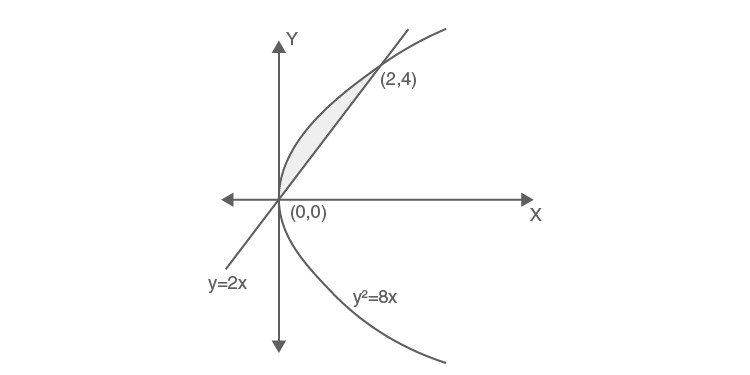
11. The area of the region bounded by the curve y² = 8x and the line y = 2x is

a. (8/3) sq. units
b. (16/3) sq. units
c. (4/3) sq. units
d. (3/4) sq. units

Solution:

Answer: (c)

Solving y² = 8x and y = 2x, we get

(x,y) = (0,0), (2,4)

So, area bounded by the curve is

12. The value of

\int_{- π / 2}^{π / 2} \frac{c o s x}{1 + e^{x}} d x

a. -2
b. 2
c. 0
d. 1

Solution:

Answer: (d)

13. The order of the differential equation obtained by eliminating arbitrary constants in the family of curves c₁y = (c₂+c₃)e ^x+c4 is

a. 4
b. 1
c. 2
d. 3

Solution:

Answer: (b)

$y = [(\frac{C_{2} + C_{3}}{C_{1}}) e^{C_{4}}] e^{x} = A e^{x},$

where A =
$(\frac{C_{2} + C_{3}}{C_{1}}) e^{C_{4}}$

Order = Number of independent arbitrary constants = 1

14. The general solution of the differential equation x²dy-2xydx = x⁴cos x dx is

a. y = cos x+cx²
b. y = x² sin x+cx²
c. y = x²sin x+cx
d. y = sin x+cx²

Solution:

Answer: (b)

x²dy – 2xydx = x⁴ cosx dx

$\frac{d y}{d x} = \frac{x^{4} c o s x + 2 x y}{x^{2}}$

⇒dy/dx – 2y/x =x² cos x

I.F. = e^{∫-2/x dx} = e^{-2 logx} = 1/x²

Therefore, the general solution is

$y (\frac{1}{x^{2}}) = \int \frac{1}{x^{2}} (x^{2} c o s x) d x = s i n x + c$

∴y = x² (sinx+c)

= x² sinx+cx²

15. The area of the region bounded by the line y = 2x+1, x- axis and the ordinates x = -1 and x = 1 is

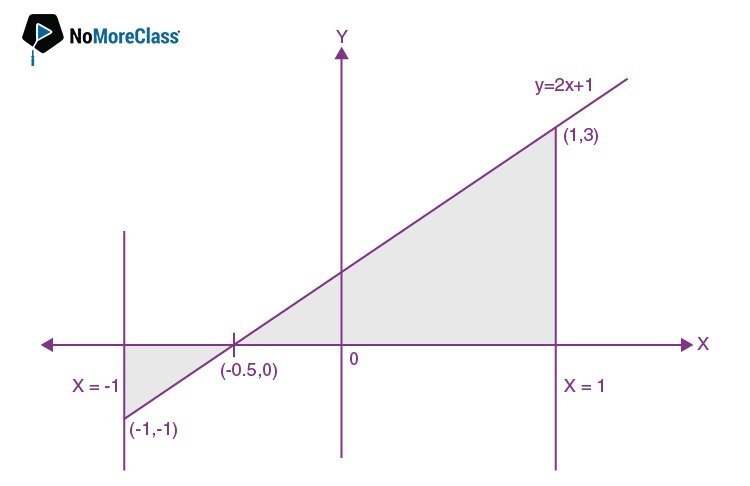
a. 5
b. 9/4
c. 2
d. 5/2

Solution:

Answer: (d)

Area bounded by y = 2x+1 with x- axis

= (1/2) (1/2)(1) +(1/2) (3/2)(3) = 5/2 sq. units.

16. The two vectors

and
$\hat{i} + 3 \hat{j} + 5 \hat{k}$
represent the two sides
$\vec{A B}$
and
$\vec{A C}$
respectively of a ∆ABC. The length of the median through A is

a. 14
b. 14/2
c. 14
d. 7

Solution:

Answer: (a)

17. If

$\vec{a}$

and
$\vec{b}$
are unit vectors and θ is the angle between
$\vec{a}$
and
$\vec{b}$
, then sin(θ/2)is

Solution:

Answer: (d)

18. The curve passing through the point (1, 2) given that the slope of the tangent at any point (x,y) is 2x/y represents

a. Hyperbola
b. Circle
c. Parabola
d. Ellipse

Solution:

Answer: (a)

Given,
$\frac{d y}{d x} = \frac{2 x}{y}$

⇒ ydy = 2xdx

⇒ ∫ydy = ∫2x dx

⇒ y²/2 = x²+A, where A is a constant.

The above equation represents a hyperbola.

19. If

${| \vec{a} \times \vec{b} |}^{2} + {| \vec{a} \cdot \vec{b} |}^{2}$

and
$| \vec{a} |$
= 6, then
$| \vec{b} |$
is equal to

a. 4
b. 6
c. 3
d. 2

Solution:

Answer: (d)

20. The point(1,-3,4) lies in the octant

a. Eighth
b. Second
c. Third
d. Fourth

Solution:

Answer: (d)

Signs of x-coordinate, y-coordinate and z-coordinate are +,-,+ respectively.

∴(1,-3,4) lies in the fourth octant.

21. If the vector

$2 \hat{i} - 3 \hat{j} + 4 \hat{k}$

2 \hat{i} + \hat{j} - \hat{k}

,
$2 \hat{i} + \hat{j} - \hat{k}$
and
$λ \hat{i} - \hat{j} + 2 \hat{k}$
are coplanar, then the value of λ is

a. 5
b. 6
c. -5
d. -6

Solution:

Answer: (b)

Given vectors are coplanar.

⇒
$| \begin{matrix} 2 & - 3 & 4 \\ 2 & 1 & - 1 \\ λ & - 1 & 2 \end{matrix} | = 0$

⇒ 2(1) + 3(4 + λ) + 4(-2 – λ) = 0

⇒ 2 + 12 + 3λ – 8 – 4λ = 0

⇒ λ = 6

22. The distance of the point (1,2,-4) from the line (x-3)/2 = (y-3)/3 = (z+5)/6 is

a. √293/49
b. 293/7
c. √293/7
d. 293/49

Solution:

Answer: (c)

Let
$\frac{x - 3}{2} = \frac{y - 3}{3} = \frac{z + 5}{6} = t$

⇒ (x, y, z) = (2t + 3, 3t + 3, 6t – 5)

∴ d.r.’s of the line perpendicular to
$\frac{x - 3}{2} = \frac{y - 3}{3} = \frac{z + 5}{6}$
and joining (2t+3,3t+3,6t-5)

and (1,2,-4) is (2t+2,3t+1,6t-1)

∴2(2t+2)+3(3t+1)+6(6t-1)=0

⇒t = -1/49

∴ Distance =
$\sqrt{(2 t + 2)^{2} + (3 t + 1)^{2} + (6 t - 1)^{2}} = \sqrt{49 t^{2} + 2 t + 6}$

=
$\sqrt{\frac{1}{49} - \frac{2}{49} + 6} = \frac{\sqrt{293}}{7}$

23. The sine of the angle between the straight line (x-2)/3 = (3-y)/(-4) = (z-4)/5 and the plane

a. √2/10
b. 3/√50
c. 3/50
d. 4/5√2

Solution:

Answer: (a)

Given line is
$\frac{x - 2}{3} = \frac{y - 3}{4} = \frac{z - 4}{5}$

and plane is 2x-2y+z = 5

24. If a line makes an angle of π/3 with each of x and y-axis, then the acute angle made by z-axis is

a. π/2
b. π/4
c. π/6
d. π/3

Solution:

Answer: (b)

Given, α = β = π/3

Let acute angle made by z- axis be γ.

Then,
$c o s^{2} α + c o s^{2} β + c o s^{2} γ$

⇒
$(\frac{1}{2})^{2} + (\frac{1}{2})^{2} + c o s^{2} γ = 1$

⇒
$\frac{1}{4} + \frac{1}{4} + c o s^{2} γ = 1 \Rightarrow c o s^{2} γ = \frac{1}{2}$

⇒
$c o s γ = \pm \frac{1}{\sqrt{2}} \Rightarrow γ = \frac{π}{4}$

[∵γ is acute]

25. Corner points of the feasible region determined by the system of linear constraints are (0,3),(1,1) and (3,0). Let z = px+qy, where p,q>0. Condition on p and q so that the minimum of z occurs at (3,0) and (1,1) is

a. p = q
b. p = 2q
c. p = q/2
d. p = 3q

Solution:

Answer: (c)

Given corner points are (0,3),(1,1),(3,0)

z = px + qy

At (3,0), z = 3p

At (1,1), z = p + q

It is given that the minimum of z occurs at (3, 0) and (1, 1)

⇒ 3p = p + q

⇒ 2p = q

⇒ p = q/2

26. The feasible region of an LPP is shown in the figure. If Z = 11x+7y, then the maximum value of Z occurs at

a. (3,2)
b. (0,5)
c. (3,3)
d. (5,0)

Solution:

Answer: (a)

y-intercept of x+y = 5 is (0,5)

y-intercept of x+3y = 9 is (0,3)

The intersection point of x+y = 5 and x+3y = 9 is (3,2)

Therefore, the corner points are (0,5),(0,3),(3,2)

At (0,5), Z = 35

At (0,3), Z = 21

At (3,2), Z = 47

So, Z_max= 47 at (3,2).

27. A die is thrown 10 times, the probability that an odd number will come up atleast one time is

a. 1013/1024
b. 1/1024
c. 1023/1024
d. 11/1024

Solution:

Answer: (c)

Given n = 10

Probability of odd number, p = ½

∴q=1/2

Required probability = P(X≥1)

=1-P(X=0)

=
$1 -^{10} C_{0} (1 / 2)^{10 - 0} (1 / 2)^{0}$

= 1 – 1/2¹⁰

= 1 – 1/1024

= 1023/1024

28. If A and B are two events such that P(A) = 1/3, P(B) = 1/2 and P(A∩B) = 1/6, then P(A’|B) is

a. 1/12
b. 2/3
c. 1/3
d. 1/2

Solution:

Answer: (b)

Given P(A) = 1/3, P(B) = 1/2, P(A ∩ B) = 1/6

So, P(A’|B) = 1 – P(A|B)

=
$1 - \frac{P (A \cap B)}{P (B)}$

= 1 – 1/3

= 2/3

29. Events E₁ and E₂ form a partition of the sample space S.A is any event such that P(E₁) = P(E₂) = 1/2, P(E₂│A) = 1/2 and (A│E₂) = 2/3 . Then P(E₁│A) is

a. 1/4
b. 1/2
c. 2/3
d. 1

Solution:

Answer: (b)

Let P(A|E₁) = x

By Bayes’ theorem,

30. The probability of solving a problem by three persons A,B and C independently is 1/2,1/4 and 1/3 respectively. Then the probability that the problem is solved by any two of them is

a. 1/8
b. 1/12
c. 1/4
d. 1/24

Solution:

Answer: (c)

Required probability = P(A’BC) + P(AB’C) + P(ABC’)

= 1/2 × 1/4 × 1/3 + 1/2 × 3/4 × 1/3 + 1/2 × 1/4 × 2/3

= 1/24 + 1/8 + 1/12

=(1+3+2)/24

= 1/4

31. If n(A) = 2 and total number of possible relations from set A to set B is 1024, then n(B) is.

a. 5
b. 512
c. 20
d. 10

Solution:

Answer: (a)

n(A) = 2

Given, 2^{(n(A)⋅n(B))} = 1024

⇒(2)^(2⋅n(B))= (2)¹⁰

⇒2⋅n(B) = 10

⇒n(B) = 5

32. The value of sin² 51⁰ + sin²39⁰ is

a. cos 12⁰
b. 1
c. 0
d. sin 12⁰

Solution:

Answer: (b)

sin² 51°+sin² 39°

= cos² 39° + sin² 39°

= 1

33. If tan A+cot A=2, then the value of tan⁴ A+cot⁴ A=

a. 5
b. 2
c. 1
d. 4

Solution:

Answer: (b)

tan A + cot A = 2

⇒ (tan A + cotA )² = 4

⇒tan² A + cot² A + 2tan A cotA = 4

⇒tan² A+cot² A=2

⇒(tan² A+cot² A)²=4

⇒tan⁴ A+cot⁴ A+2 tan² A cot² A=4

⇒tan⁴ A+cot⁴ A=2

34. If A={1,2,3,4,5,6}, then the number of subsets of A which contain atleast two elements is

a. 58
b. 64
c. 63
d. 57

Solution:

Answer: (d)

Total number of subsets of A is 2^n(A) = 2⁶ = 64

Number of subsets of A which contain at least two elements is

64-( ⁶C₀ + ⁶C₁ )

= 64-(1+6)

= 57

35. If z = x + iy, then the equation |z+1| = |z-1| represents

a. y-axis
b. a circle
c. a parabola
d. x-axis

Solution:

Answer: (a)

36. The value of ¹⁶C₉+¹⁶ C₁₀– ¹⁶C₆– ¹⁶C₇is

a. ¹⁷C₂
b. 0
c. 1
d. ¹⁷C₁₀

Solution:

Answer: (b)

¹⁶C₉+ ¹⁶C₁₀– ¹⁶C₆– ¹⁶C₇

= ¹⁶C₉+ ¹⁶C₁₀– ¹⁶C₁₀– ¹⁶C₉=0 (∵ ⁿC_r = ⁿC_(n-r))

37. The number of terms in the expansion of (x+y+z)10 is

a. 110
b. 66
c. 142
d. 11

Solution:

Answer: (b)

Number of terms in the expansion of (x+y+z)¹⁰ is ^(10+3-1)C₁₀

= ¹²C₁₀=12!/(2! 10!)=66

38. If P(n) ∶2ⁿ< n!,then the smallest positive integer for which P(n)is true if

a. 5
b. 2
c. 3
d. 4

Solution:

Answer: (d)

For n = 1, 2, 3, 2ⁿ> n!

P(4) ∶ 2⁴< 4!

So, smallest positive integer, n = 4.

39. The two lines lx+my = n and l’x+m’y= n’ are perpendicular if

a. lm’ + ml’= 0
b. ll’+mm’=0
c. lm’=ml’
d. lm+l’m’=0

Solution:

Answer: (b)

Product of slopes = -1

⇒ll’ + mm’ = 0

40. If the parabola x² = 4ay passes through the point (2, 1), then the length of the latus rectum is

a. 8
b. 1
c. 4
d. 2

Solution:

Answer: (c)

x²=4ay

Given parabola passes through (2,1).

⇒ 2²= 4a

⇒ a = 1

Length of latus rectum= 4a = 4.

41. If the sum of n terms of an A.P. is given by S_n = n² + n, then the common difference of the A.P. is

a. 6
b. 4
c. 1
d. 2

Solution:

Answer: (d)

S_n= n²+n

S₁ = 1+1 = 2 = T₁

S₂= 2²+2 = 6 = T₁+T₂

∴T₂= S₂-S₁= 4

Common difference, d= T₂-T₁

= 4-2

= 2

42. The negation of the statement “For all real numbers x and y, x + y = y + x” is

a. for some real numbers x and y, x – y = y – x
b. for all real numbers x and y, x+y ≠ y+x
c. for some real numbers x and y, x+y = y+x
d. for some real numbers x and y,x+y ≠ y+x

Solution:

Answer: (d)

Negation: For some real numbers x and y, x + y ≠ y + x.

43. The standard deviation of the data 6,7,8,9,10 is

a. 10
b. 2
c. 10
d. 2

Solution:

Answer: (b)

Mean,
$\bar{x} = \frac{6 + 7 + 8 + 9 + 10}{5} = \frac{40}{5} = 8$

Standard deviation,
$σ = \sqrt{\frac{1}{5} (4 + 1 + 0 + 1 + 4)}$

Because,
$σ = \sqrt{\frac{1}{n} \sum (x_{i} - \bar{x})^{2}}$

⇒
$σ = \sqrt{\frac{10}{5}} = \sqrt{2}$

44.

$l i m_{x \to 0} (\frac{t a n x}{\sqrt{2 x + 4} - 2})$

is equal to

a. 6
b. 2
c. 3
d. 4

Solution:

Answer: (b)

45. If a relation R on the set {1,2,3} be defined by R = {(1,1)}, then R is

a. Only symmetric
b. Reflexive and symmetric
c. Reflexive and transitive
d. Symmetric and transitive

Solution:

Answer: (d)

R={(1,1)} on set {1,2,3}

Clearly, R is symmetric and transitive.

46. Let f ∶ [2, ∞] → R be the function defined by f(x) = x²-4x+5, then the range of f is

a. [5,∞)
b. (-∞,∞)
c. [1, ∞)
d. (1,∞)

Solution:

Answer: (c)

f(x) = (x-2)² + 1 ≥ 1, ∀ x ∈ [2,∞)

f_min = 1 at x = 2

∴ Range of f is [1, ∞)

47. If A,B,C are three mutually exclusive and exhaustive events of an experiment such that P(A) = 2P(B) = 3P(C), then P(B) is equal to

a. 4/11
b. 1/11
c. 2/11
d. 3/11

Solution:

Answer: (d)

Given, P(A) = 2P(B) = 3P(C)

⇒ P(C) = 2/3 P(B)

Since A,B,C are three mutually exclusive and exhaustive events

∴P(A)+P(B)+P(C) = 1

⇒ P(B) = 3/11

48. The domain of the function defined by f(x) = cos^-1 √(x-1)is

a. [0,1]
b. [1,2]
c. [0,2]
d. [-1,1]

Solution:

Answer: (b)

For f to be defined,

x -1 ≥ 0 and -1 ≤ √(x-1) ≤ 1

⇒ x ≥ 1 and 0 ≤ x-1 ≤ 1

⇒ x ≥ 1 and 1 ≤ x ≤ 2

⇒ x ∈ [1,2]

Hence, domain of f is [1, 2].

49. The value of

$c o s (s i n^{- 1} \frac{π}{3} + c o s^{- 1} \frac{π}{3})$

a. Does not exist
b. 0
c. 1
d. -1

Solution:

Answer: (a)

π/3 ∉ [-1,1] which is the domain of sin^-1 x, cos^-1 x

So, cos(sin^-1 π/3 + cos^-1 π/3) does not exist.

$(\begin{matrix} 0 & 0 & 1 \\ 0 & 1 & 0 \\ 1 & 0 & 0 \end{matrix})$

Answer: (d)

, then A4 is equal to

a. 4A
b. A
c. 2A
d. I

Solution:

Answer: (d)

51. If A = {a,b,c}, then the number of binary operations on A is

a. 3⁹
b. 3
c. 3⁶
d. 3³

Solution:

Answer: (a)

A = {a,b,c}

n(A) = 3

Number of binary operations is n(A)^{(n(A))^2} = 3^{(3^2)} = 3⁹

52. If

(2132)A=(1001)

, then the matrix A is

a.
(2−132)
b.
(2132)
c.
(2−1−32)
d.
(−213−2)

Solution:

Answer: (c)

53. If f(x) =

$| \begin{matrix} x^{3} - x & a + x & b + x \\ x - a & x^{2} - x & c + x \\ x - b & x - c & 0 \end{matrix} |$

, then

a. f(-1) = 0
b. f(1) = 0
c. f(2) = 0
d. f(0) = 0

Solution:

Answer: (d)

$f (0) = | \begin{matrix} 0 & a & b \\ - a & 0 & c \\ - b & - c & 0 \end{matrix} |$

f(0) is the determinant of skew-symmetric matrix of order 3 (odd).

∴f(0) = 0.

54. If A and B are square matrices of same order and B is a skew symmetric matrix, then A’BA is

a. Skew symmetric matrix
b. Symmetric matrix
c. Null matrix
d. Diagonal matrix

Solution:

Answer: (a)

B is a skew symmetric matrix.

⇒ B’ = -B

Now, (A’BA)’ = A’B'(A’)’

= A'(-B)A

= -(A’ BA)

Hence, A’BA is a skew symmetric matrix.

55. If A is a square matrix of order 3 and |A| = 5, then |A adj A| is

a. 625
b. 5
c. 125
d. 25

Solution:

Answer: (c)

|A adj A| = |A| |adj A|

= |A||A|^3-1

= 5 5²

= 125

56. If

$f (x) = {\begin{matrix} \frac{1 - c o s k x}{x s i n x} & i f x \neq 0 \\ \frac{1}{2} & i f x = 0 \end{matrix}$

is continuous at x = 0, then the value of K is

a. ±1
b. ±1/2
c. 0
d. ±2

Solution:

Answer: (a)

Given, f is continuous at x = 0.

57. If a₁,a₂,a₃,……,a₉are in A.P. then the value of

|a1a2a3a4a5a6a7a8a9|

a. 1
b. (9/2)(a₁+a₉)
c. a₁+a₉
d. log_e(log_ee)

Solution:

Answer: (d)

59. If

$f (x) = s i n^{- 1} (\frac{2 x}{1 + x^{2}})$

, then f’(√3)is

a. -1/√3
b. -1/2
c. 1/2
d. 1/√3

Solution:

Answer: (c)

60. The right hand and left limit of the function

$f (x) = {\begin{matrix} \frac{e^{1 / x} - 1}{e^{1 / x} + 1} & i f x \neq 0 \\ 0 & i f x = 0 \end{matrix}$

are respectively

a. -1 and 1
b. 1 and 1
c. 1 and -1
d. -1 and -1

Solution:

Answer: (c)

Talk to our expert

+91 74112 69409

Request call back

KCET 2020 MATHEMATICS QUESTION PAPER

Overview of the KCET Exam

Importance of Mathematics Section in KCET

Format and Structure of KCET 2020 Mathematics Paper

Difficulty Level of the Questions

Key Topics Covered in the Mathematics Section

Algebra

Trigonometry

Calculus

Geometry

KCET 2020 MATHEMATICS QUESTION PAPER

Talk to our expert

Contact

Company

Useful links

Support

KCET 2020 MATHEMATICS QUESTION PAPER

Overview of the KCET Exam

Importance of Mathematics Section in KCET

Format and Structure of KCET 2020 Mathematics Paper

Difficulty Level of the Questions

Key Topics Covered in the Mathematics Section

Algebra

Trigonometry

Calculus

Geometry

KCET 2020 MATHEMATICS QUESTION PAPER

Talk to our expert

Contact

Company

Useful links

Support

Login with your site account

Login with your site account